Doob–Dynkin lemma
In mathematics, the Doob–Dynkin lemma, named after Joseph Doob and Eugene Dynkin, is a statement in probability theory that characterizes the situation when one random variable is a function of another, in terms of measurability and 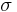 algebras.
algebras.
Statement of the lemma
Let 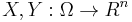 and
and 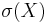 be the
be the 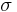 algebra generated by
algebra generated by  . Then
. Then 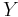 is
is 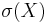 measurable if and only if
measurable if and only if 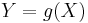 for some Borel measurable function
for some Borel measurable function 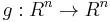 .
.
References
- A. Bobrowski: Functional analysis for probability and stochastic processes: an introduction, Cambridge University Press (2005), ISBN 0-521-83166-0
- M. M. Rao, R. J. Swift : Probability Theory with Applications, Mathematics and Its Applications, Band 582, Springer-Verlag (2006), ISBN 0-387-27730-7